Baylee tried to solve the differential equation $$\frac{d y}{d x}=\frac{e^{x}}{y^{2}}$$. This is her work: $$\quad \quad\frac{d y}{d x}=\frac{e^{x}}{y^{2}} .$$ Step 1:- $$\int y^2 d y=\int e^x d x$$ Step 2:-$$\frac{y^3}{3}=e^x$$ Step 3:-$$y^3=3 e^x$$ Step 4:![](http://admin.getprephub.com/media/editor/Screenshot%202022-11-07%20103638_20221107050648129632.png) Is Baylee's work correct? If not, what is her mistake?

Here, $$\int y^{2} d y=\int e^{x} d x$$ or, $$\frac{y^{3}}{3}=e^{x}+c$$ So, step 2 is Wrong.

Prephub

Question

Difficulty Level:

Easy

MCQ

Baylee tried to solve the differential equation $\frac{d y}{d x}=\frac{e^{x}}{y^{2}}$ .
This is her work:
$\quad \quad\frac{d y}{d x}=\frac{e^{x}}{y^{2}} .$

Step 1:- $\int y^2 d y=\int e^x d x$

Step 2:- $\frac{y^3}{3}=e^x$

Step 3:- $y^3=3 e^x$

Step 4: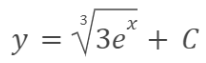
Is Baylee’s work correct? If not, what is her mistake?

Select all that apply :

Step 2 is incorrect. The right hand side of the equation should be $e^{x}+c$ .

Baylee’s work is correct.

Step 1 is incorrect. The separation of variables was not done properly.

Step 4 is incorrect. The right hand side of the equation should be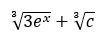

0 Claps

－ Create Free Account

Create a free account to view solution to this Question

By signing up, you accept PrepHub’sterms of serviceand dataprivacy policy.

－ Create Free Account

Create a free account to view solution to this Question

By signing up, you accept PrepHub’sterms of serviceand dataprivacy policy.